How to solve indefinite integral of square root of x divided by x rise to 5 by dx ?
Short answer: indefinite integral of square root of x divided by x rise to 5 by dx is (-2/(7x^3√x))+C. ∫ (√x)/x^5)dx is not particulary hard integral. You can solve it in 11 easy steps. We will walk you through and explain everything. Let's start.
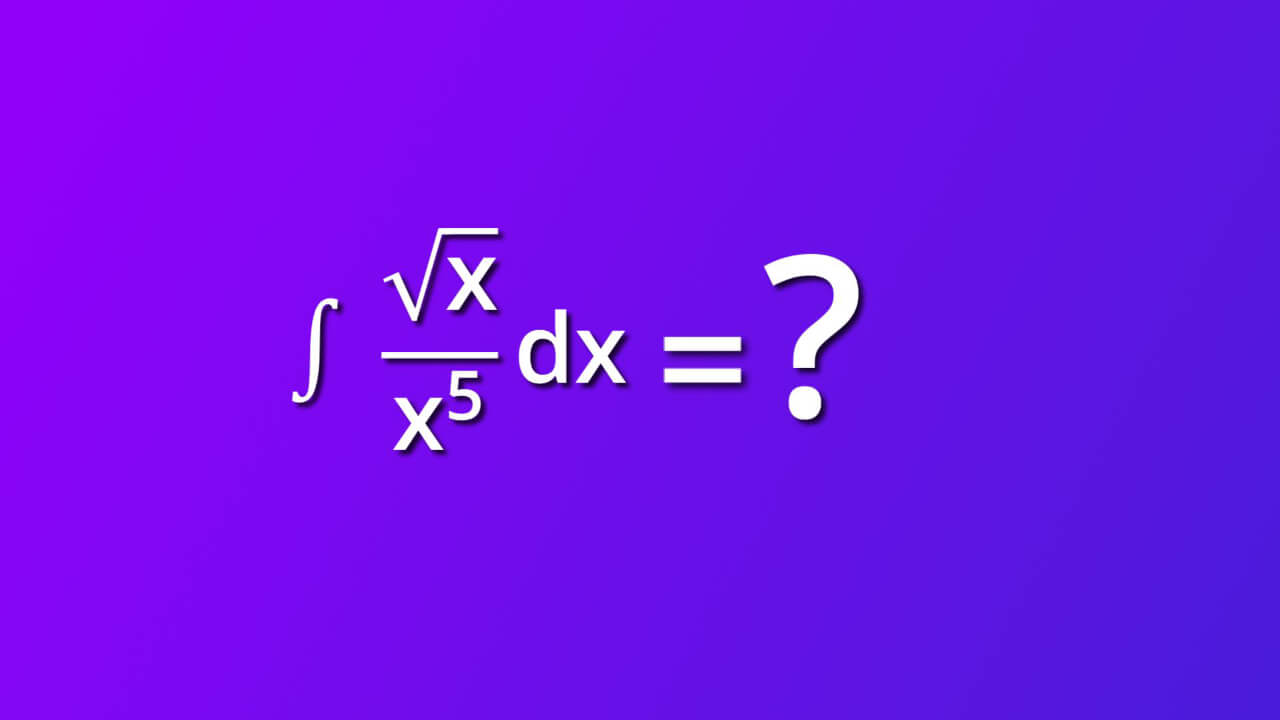
Table of contents
- Required assumtions
- Step by step solution of ∫ (√x)/x^5)dx
- What is indefinite integral of square root of x divided by x rise to 5 by dx?
- Full video how to solve ∫ (√x)/x^5)dx
- Dictionary
- Want to solve next one?
Required assumtions
Usually, we have some additional info about function f of (x). In our case:
- f(x) belongs to real numbers
- f(x) is integrable in that domain
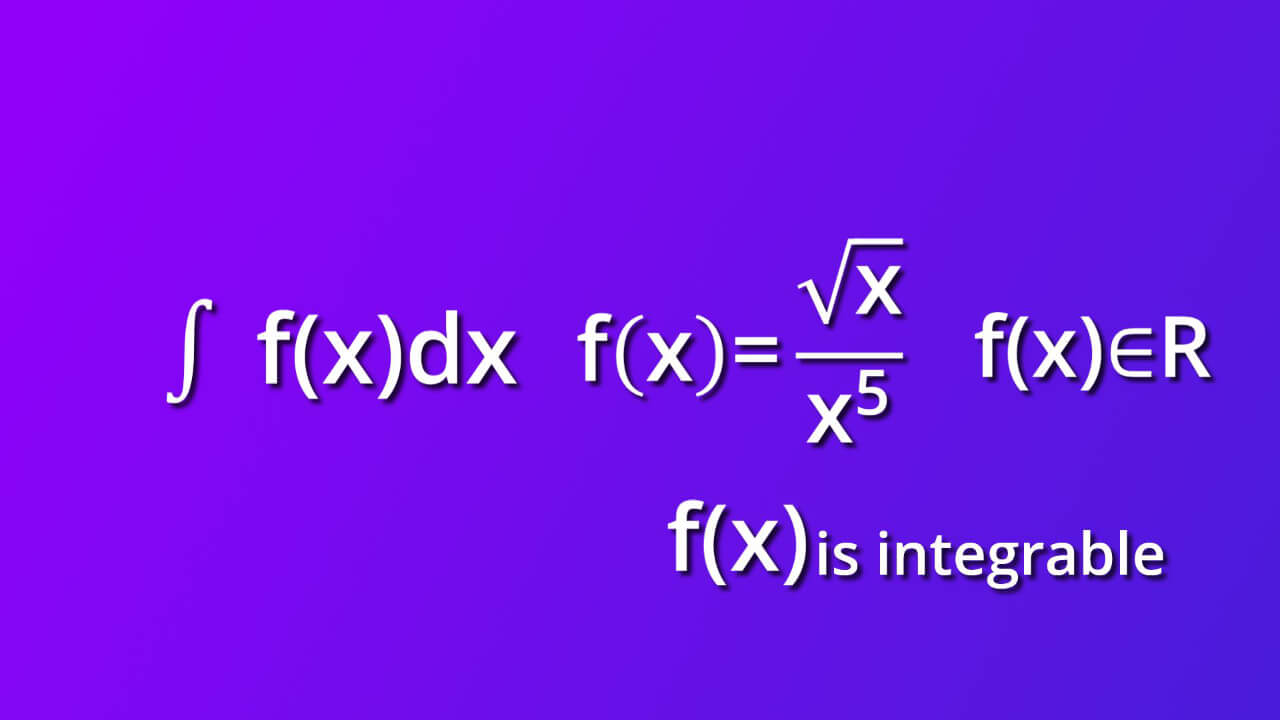
Step by step solution of ∫ (√x)/x^5)dx
We will solve ∫ (√x)/x^5)dx in 11 easy steps. Let's get started
Step 1
From symbol dx we know that differential of variable x indicates that the variable of integration is x. Let’s rewrite integrand - function inside the integral without fraction- knowing that n root of u is equal to u rise to 1 divided by n.
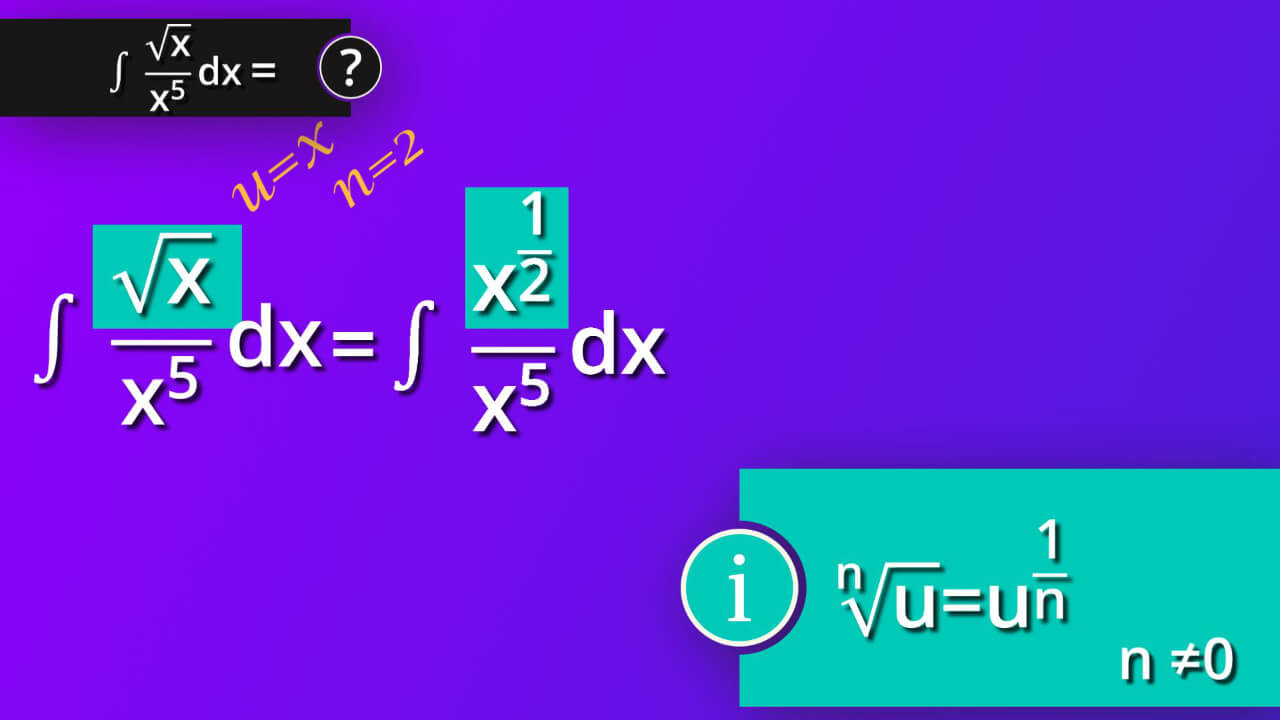
Step 2
In our case u is equal to x and n is equal to 2. Then we may divide numerator by denominator using formula u rise to n divided by u rise to m is equal to u rise to n minus m. In or case u is equal to x, n is equal to a half, m is equal to 5.

Step 3
Now we have integral of x rise to half minus 5 by dx.

Step 4
So, in short it is integral of x rise to -4 and a half by dx. After our modifications we may directly use formula, which says integral of u rise to n by du is equal to u rise to n+1 divided by n+1. In our case u is equal to x, n is equal to -4 and a half.

Step 5
Thus, we have x rise to -4 and a half plus 1 divided by -4 and a half plus one. We must also add constant C belonging to the set of real numbers because our solution is not a single function but a whole class of functions.

Step 6
So, let’s change -4 and a half plus 1 into improper fractions of the same denominator 2 and we may add their numerators. -9 plus 2 is equal to -7 and our denominator is 2.
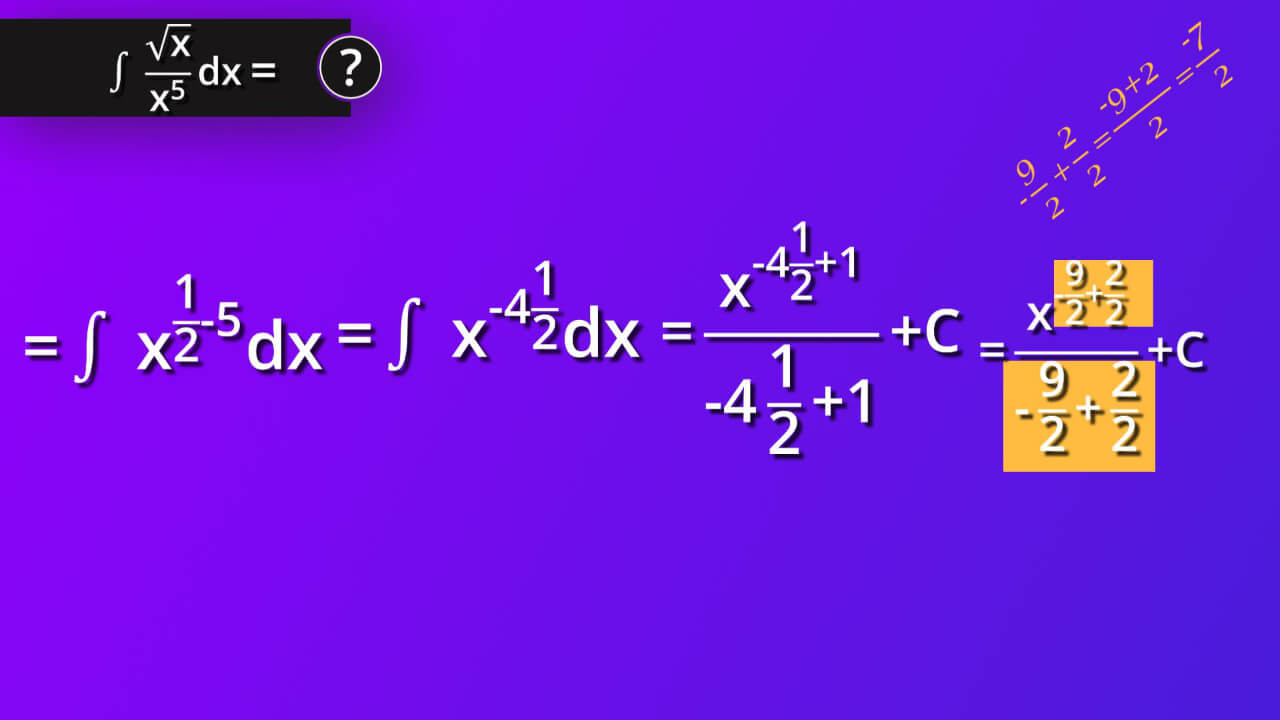
Step 7
Just to be thorough we may put this into our equation.

Step 8
Then we have x rise to -7 seconds and in denominator we have also -7 seconds. We remember about adding constant C during each step of modification that we do. To make it smoother we can modify further the equation by moving fraction from denominator to the numerator using formula u divided by a divided by b equals to b divided by a and multiplied by u. In our case u is equal to x, a is -7, b is 2. So we have -2 divided by 7 x rise to -7 seconds.
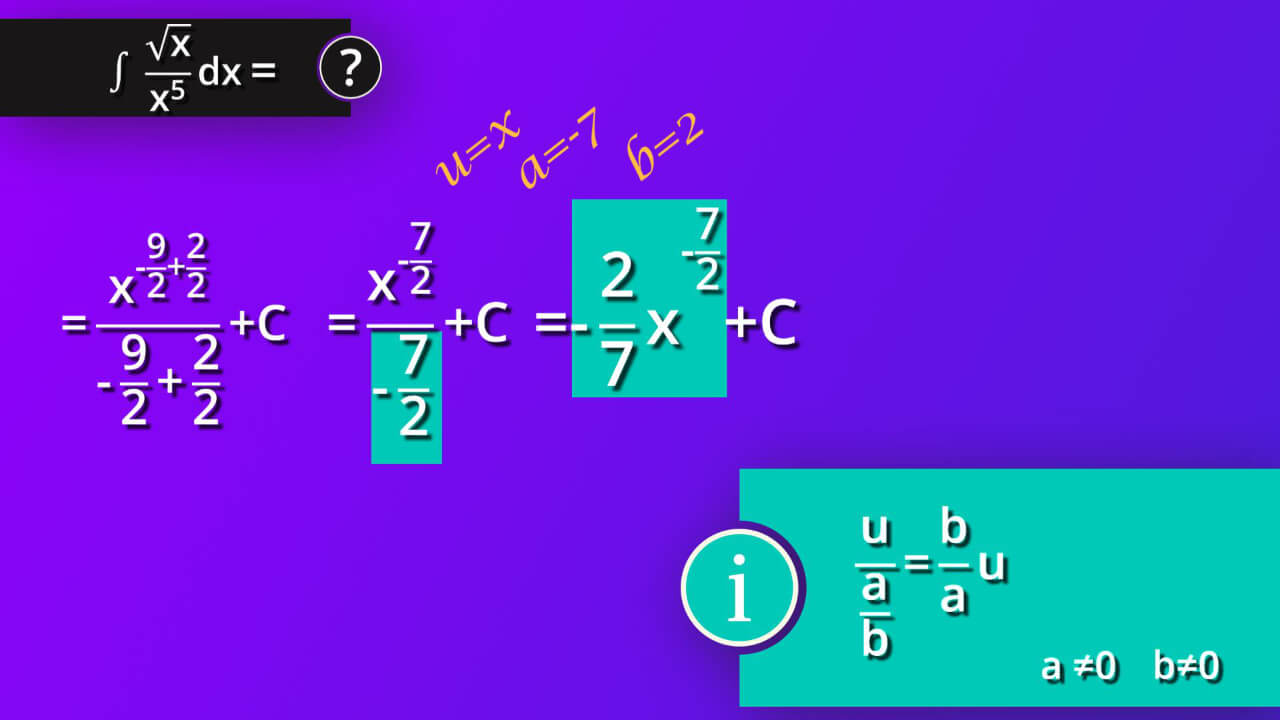
Step 9
We may also move x rise to -7 seconds to denominator knowing that u rise to -n equals 1 divided by u rise to n.
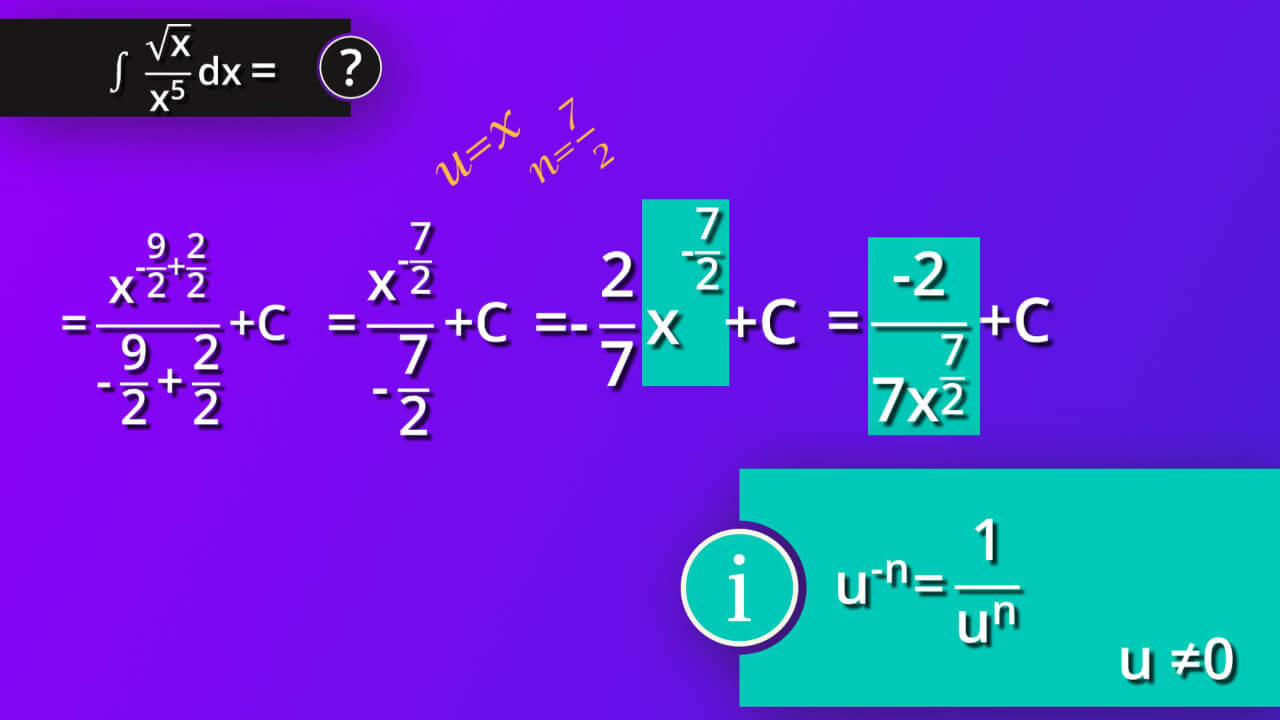
Step 10
And now we have -2 divided by 7 multiplied by x rise to 7 seconds. Also x rise to 7 seconds may be expressed smoother as it is x rise to 3 and a half so we can write it as x rise to 3 multiplied by x rise to a half. Then we have x rise to 3 multiplied by square root of x.
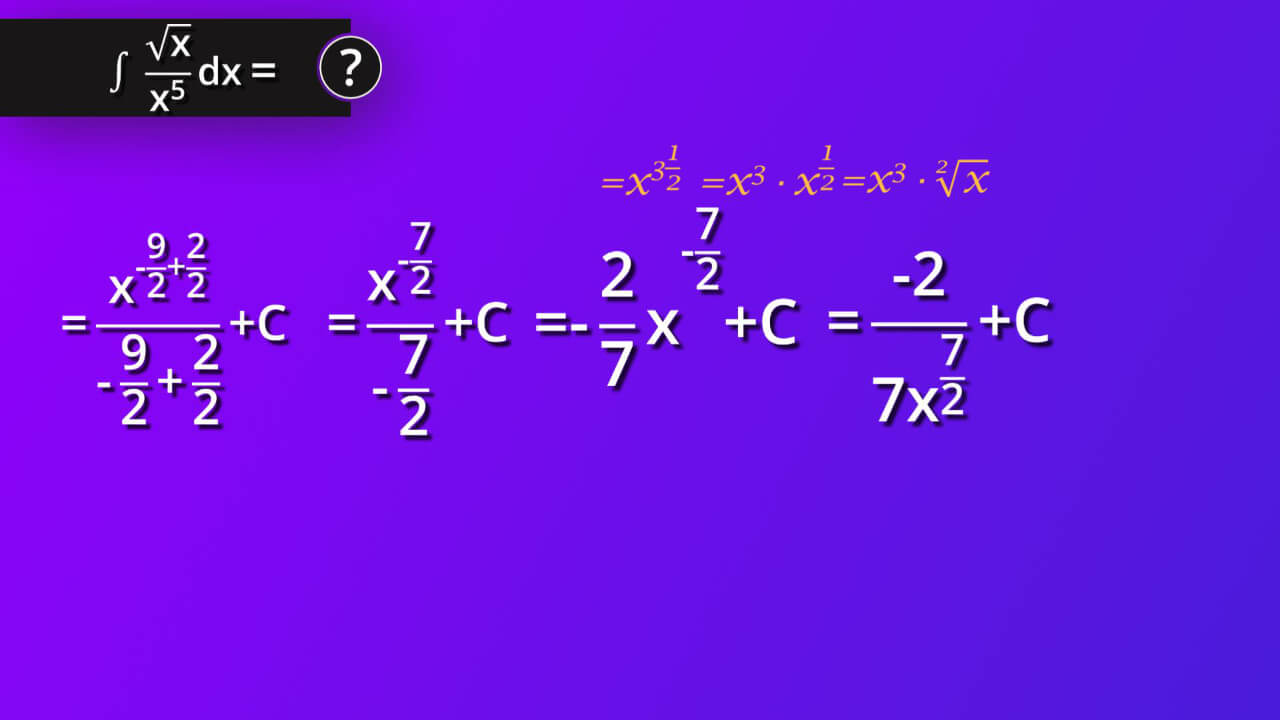
Step 11
We now put it into our equation, and we have -2 in numerator and 7 multiplied by x rise to 3 square roots of x plus C.
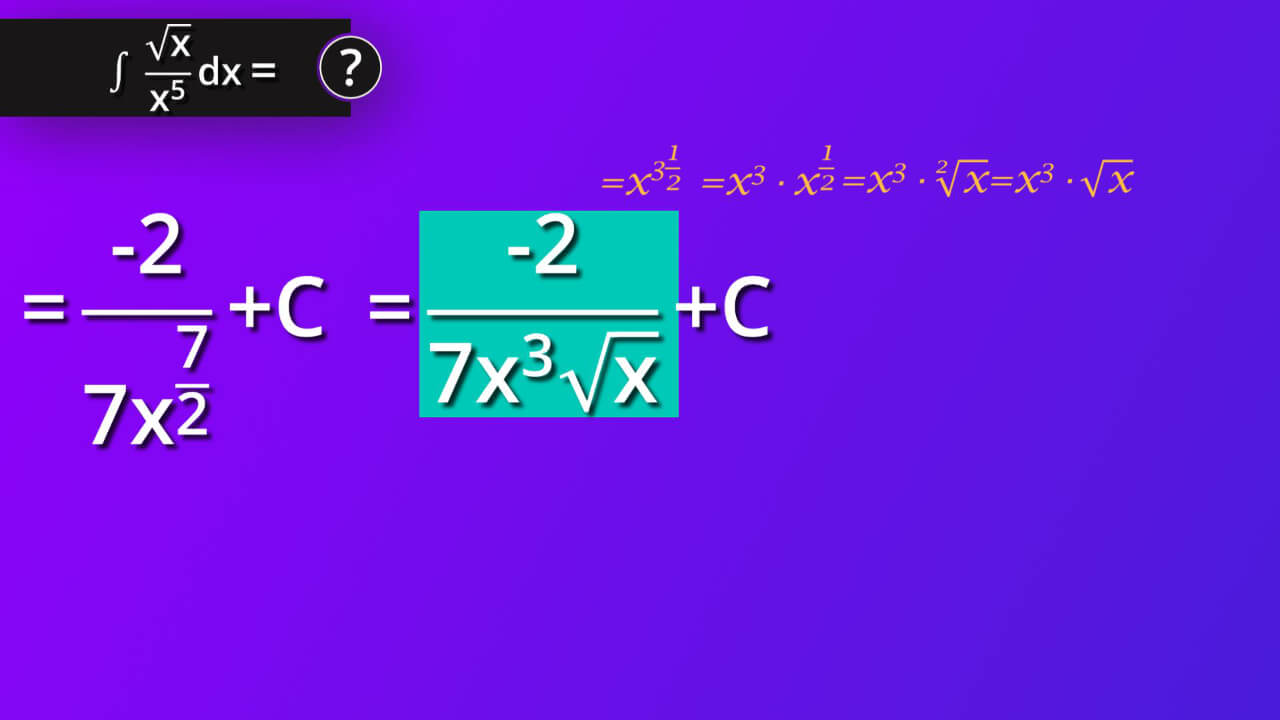
What is indefinite integral of square root of x divided by x rise to 5 by dx?
We finally did it: ∫ (√x)/x^5)dx=(-2/(7x^3√x))+C
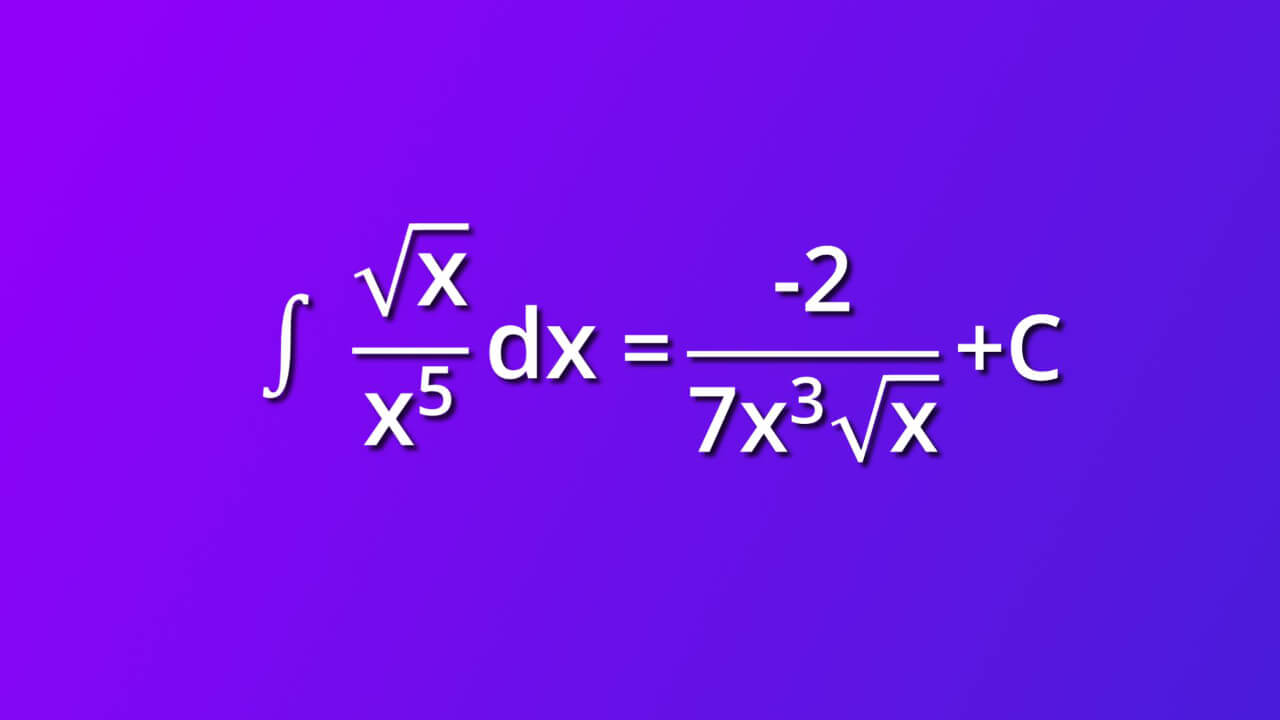
Dictionary
Integration (antidifferentiation)
Computation (process of finding) of an integral, opposite process to differentiation.Integrand
Function placed between sign of integral and differential of variable of integration e.g. $${{ \int f(x)dx}}$$,where:
$${{ \int }}$$- integration operator,
f(x) – integrand,
dx- differential of variable of integration x
Integrable function
Function that integral over its domain is finite.Indefinite integral
Represents a class of primitive functions whose derivative is the integrand e.g. $${{\int f(x)dx=F(x)+C \Leftrightarrow F’(x)=f(x)}}$$$${{C=const. }}$$,
$${{f, F,C \in R }}$$
R-real numbers
$${{\int f(x)dx}}$$ - indefinite integral of function f(x) by dx,
$${{F(x)+C}}$$ – a class of primitive functions that $${{F’(x)=f(x) }}$$,
F(x) - primitive function, usually written in capital letters,
R-real numbers.
Function
Function specified on a set X and having values in set Y is an assignment each element of set X specifically one element in set Y.$${{f: X \rightarrow Y}}$$
f-function name,
X-set of elements of function f, domain of a function f
Y-set of function values of function f, codomain of a function f
$${{x\in X, y \in Y}}$$
$${{y=f(x) f: x\rightarrow y}}$$
$${{y=y(x), }}$$
y(x)-vales of the function named y,
x-independent variable,
y-dependent variable.