How to solve indefinite integral of square root of x multiplied by cube root of x divided by x multiplied by 4th root of x by dx ?
Short answer: indefinite integral of square root of x multiplied by cube root of x divided by x multiplied by 4th root of x by dx is (12/7)(12th root of x^7)+C. ∫ ((√x∛x)/(x∜x)dx is not particulary hard integral. You can solve it in 13 easy steps. We will walk you through and explain everything. Let's start.
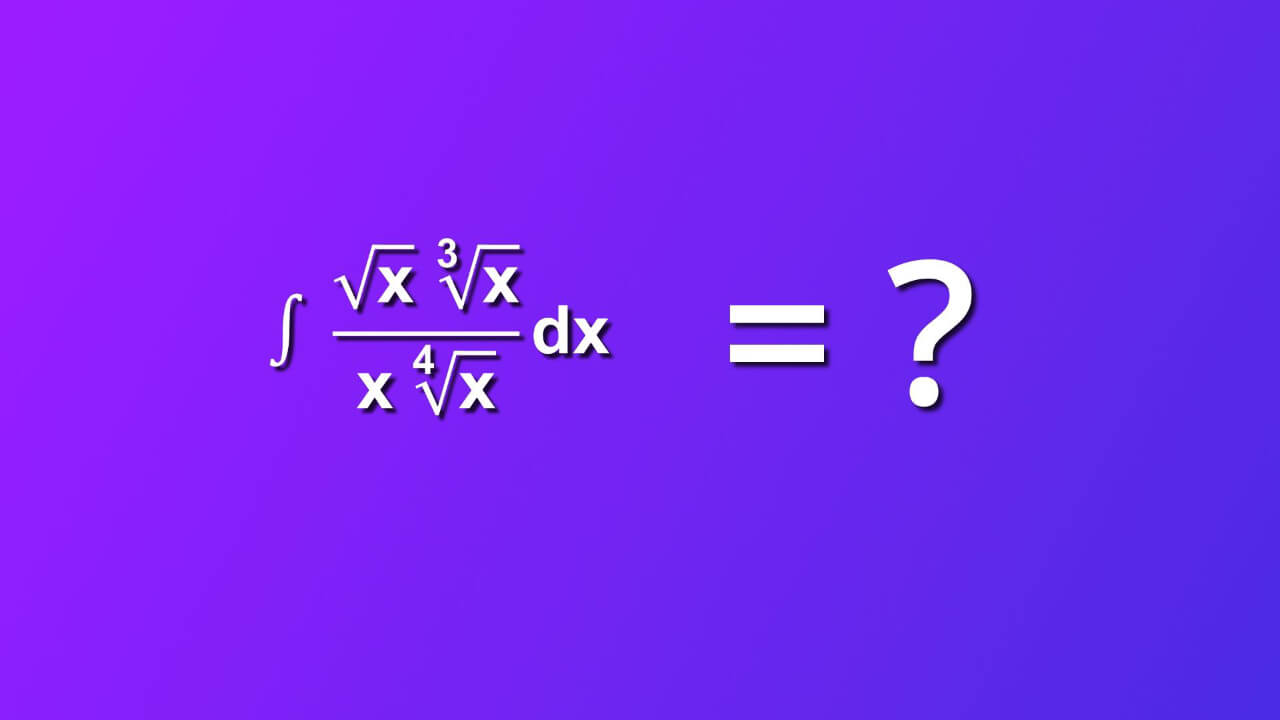
Table of contents
- Required assumtions
- Step by step solution of ∫ ((√x∛x)/(x∜x)dx
- What is indefinite integral of square root of x multiplied by cube root of x divided by x multiplied by 4th root of x by dx?
- Full video how to solve ∫ ((√x∛x)/(x∜x)dx
- Dictionary
- Want to solve next one?
Required assumtions
Usually, we have some additional info about function f of (x). In our case:
- f(x) belongs to real numbers
- f(x) is integrable in that domain
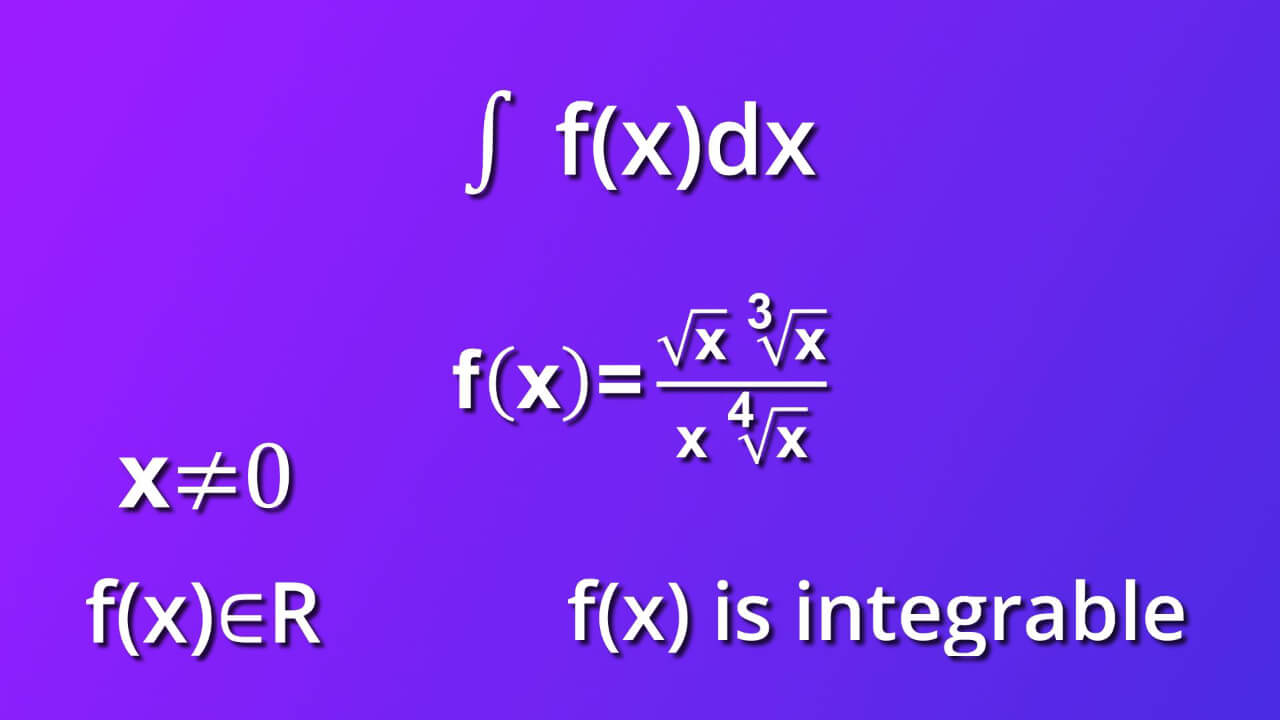
Step by step solution of ∫ ((√x∛x)/(x∜x)dx
We will solve ∫ ((√x∛x)/(x∜x)dx in 13 easy steps. Let's get started
Step 1
From symbol dx we know that differential of variable x indicates that the variable of integration is x. Let’s rewrite integrand - function inside the integral. We may apply formula which says n-th root of s equals s rise to one divided by n. First we can do it to first expression in numerator. In that case s is x and n is 2. Then, we apply it to second expression in numerator and in that case s is x but n is 3. Finally, we use it in denominator and then s is x and n is 4.
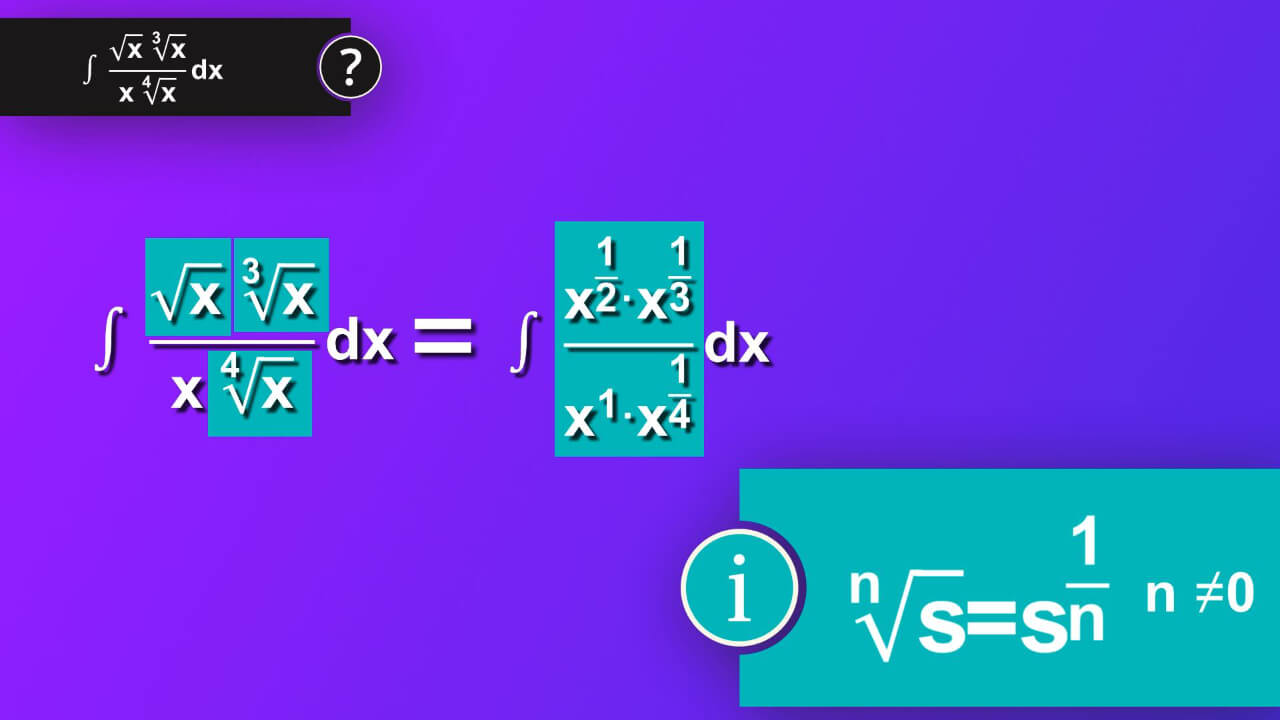
Step 2
Finally, we’ve got x rise to a half multiplied by x rise to one-third divided by x rise to 1 multiplied by x rise to one-quarter. We can express x as x rise to one using formula s is equal to s rise to one, where s is x. Now, knowing that s rise to n multiplied by s rise to m equals s rise to n plus m,
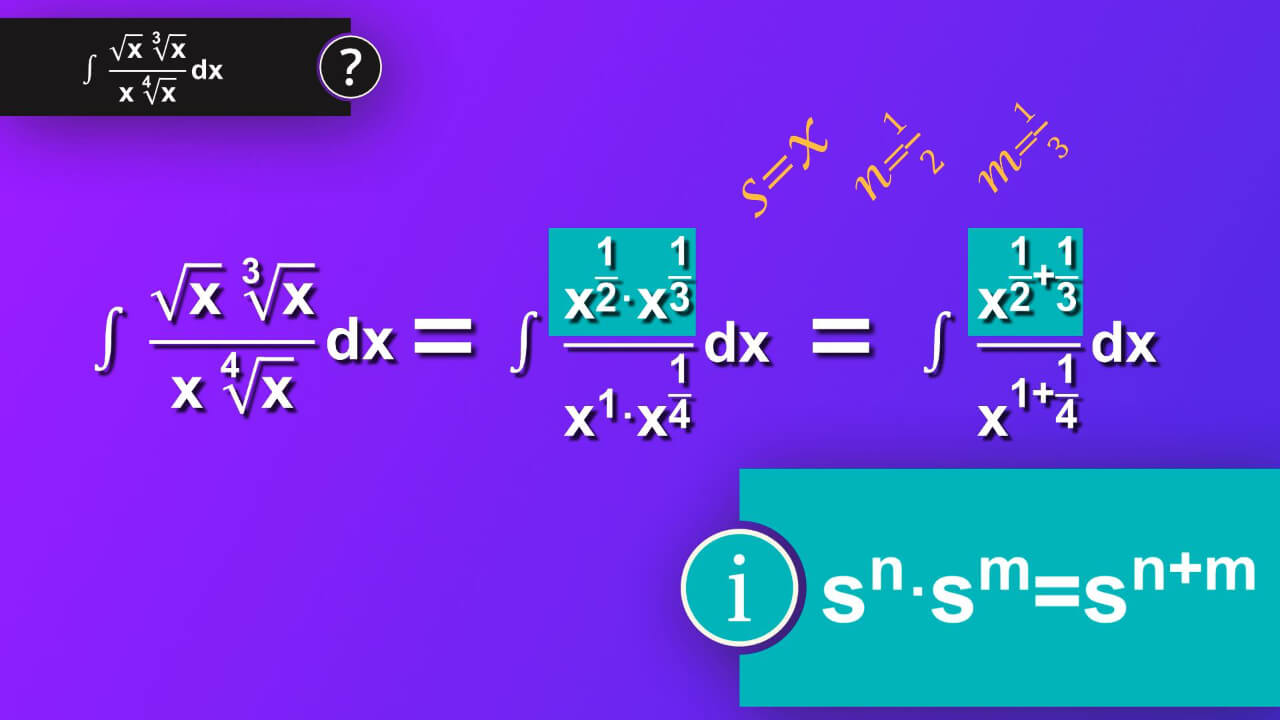
Step 3
we can rewrite numerator as x rise to a half plus one-third and denominator as x rise to one plus one-quarter.
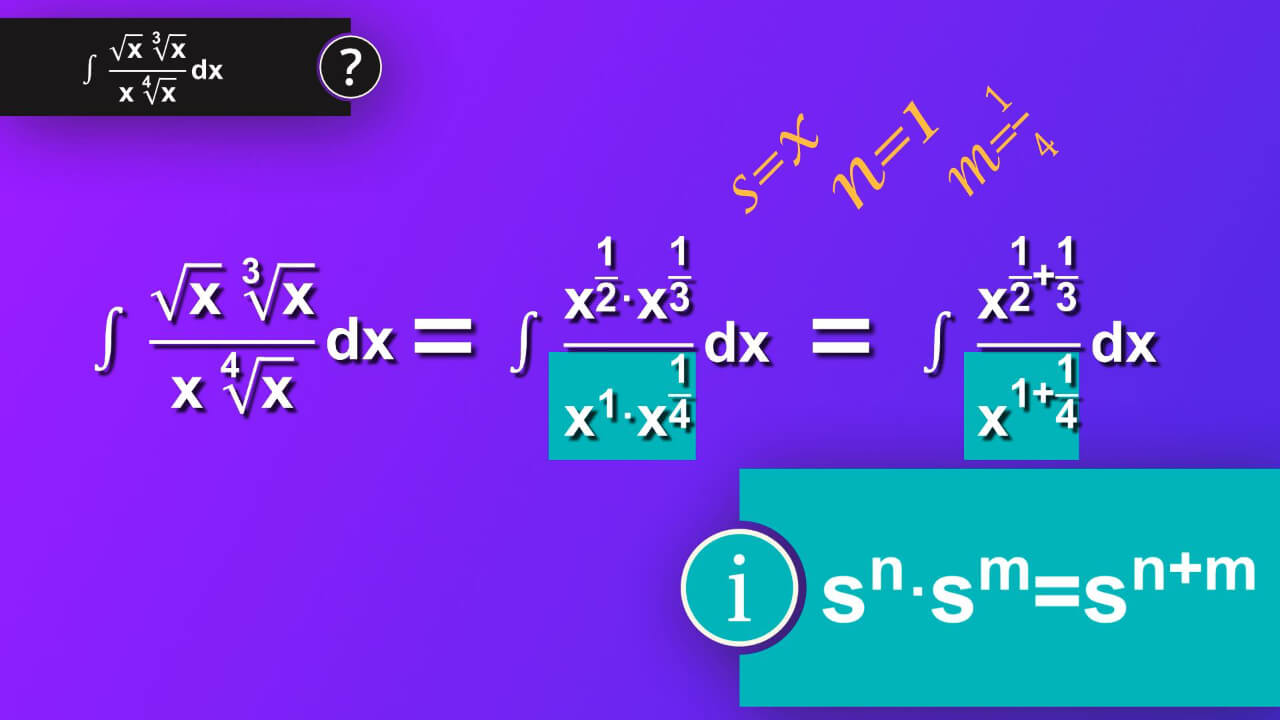
Step 4
Let’s add expressions in powers. First, we may find common denominator of 2 and 3 which is 6 and we have to multiply accordingly numerators so we’ve got 1 times 3 divided by 2 times 3 and to this we add 1 times 2 divided by 3 times 2. In that way we have common denominator 6 and in numerator we may add 3 to 2. We’ve got five-sixth and we may insert it to our main solution as x rise to five-sixth. Then we have to add one and one-quarter. We change one into four-quarters and then we have four in denominator and we may add numerators 1 plus 4. We have finally got five-quarters.
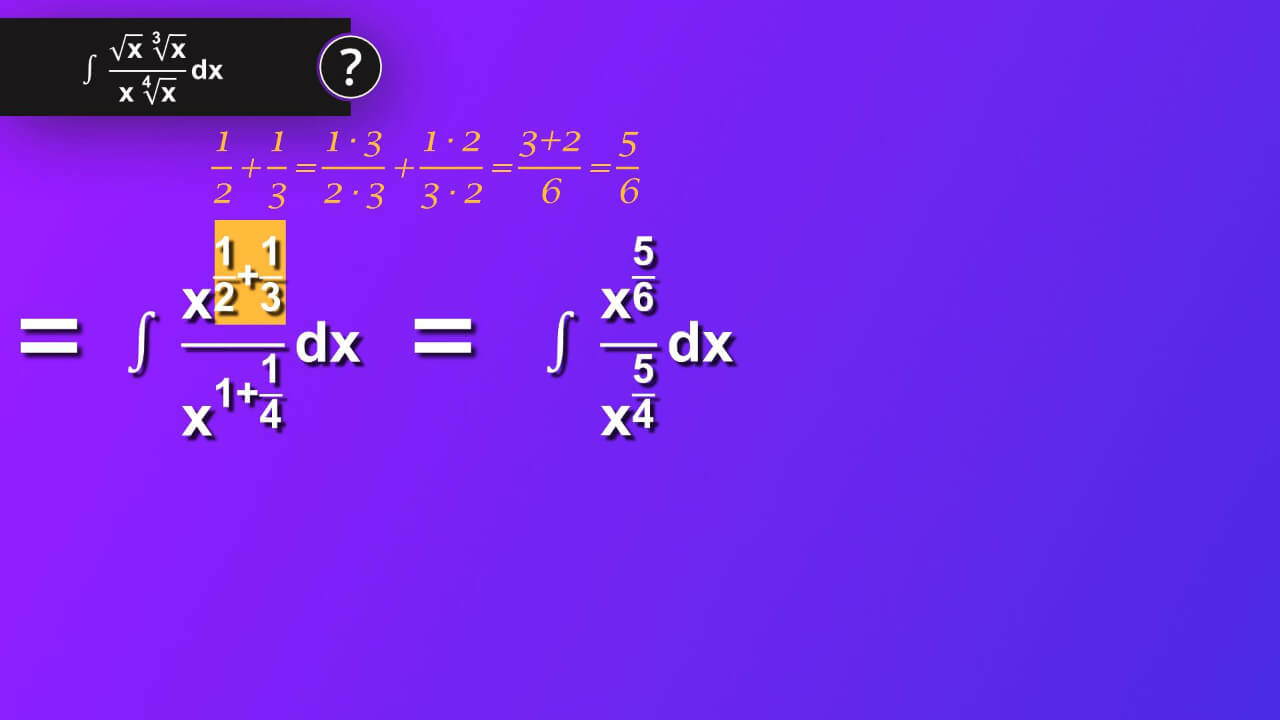
Step 5
And we may insert it to denominator of main solution. We have x rise to five-quarters in denominator. Now we can use formula s rise to n divided by s rise to m equals s rise to n minus m. In our case s equals x, n is five-sixth and m is five-quarters.

Step 6
Thus, in main solution we have x rise to five-sixth minus five-quarters.
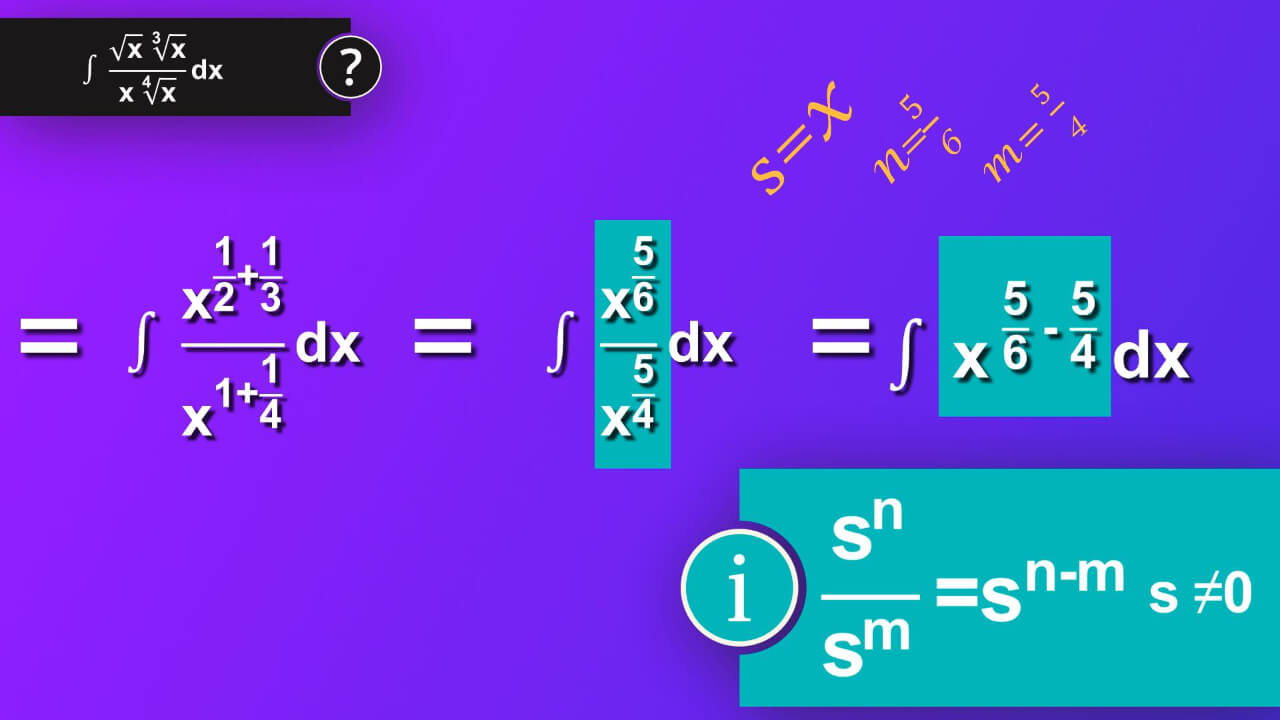
Step 7
We must subtract expression that we have in power. To do that we need common denominator and in our case it will be 12. So we must multiply numerator and denominator of five-sixth by 2 and multiply both numerator and denominator of five-quarters by 3. We’ve got then denominator 12 and in numerator we have 10 minus 15, which is minus five-twelfth.

Step 8
While we insert it to main solution, we have now indefinite integral of x rise to minus five-twelfth by dx. And after all those modifications we can easily apply formula for solving integral of s rise to n which is s rise to n plus 1 divided by n+1. We also must add constant C to make sure that we have all our solution taken into account as our solution make whole class of function not a single one. And now in our case s is equal to x and n is minus five-twelfth.
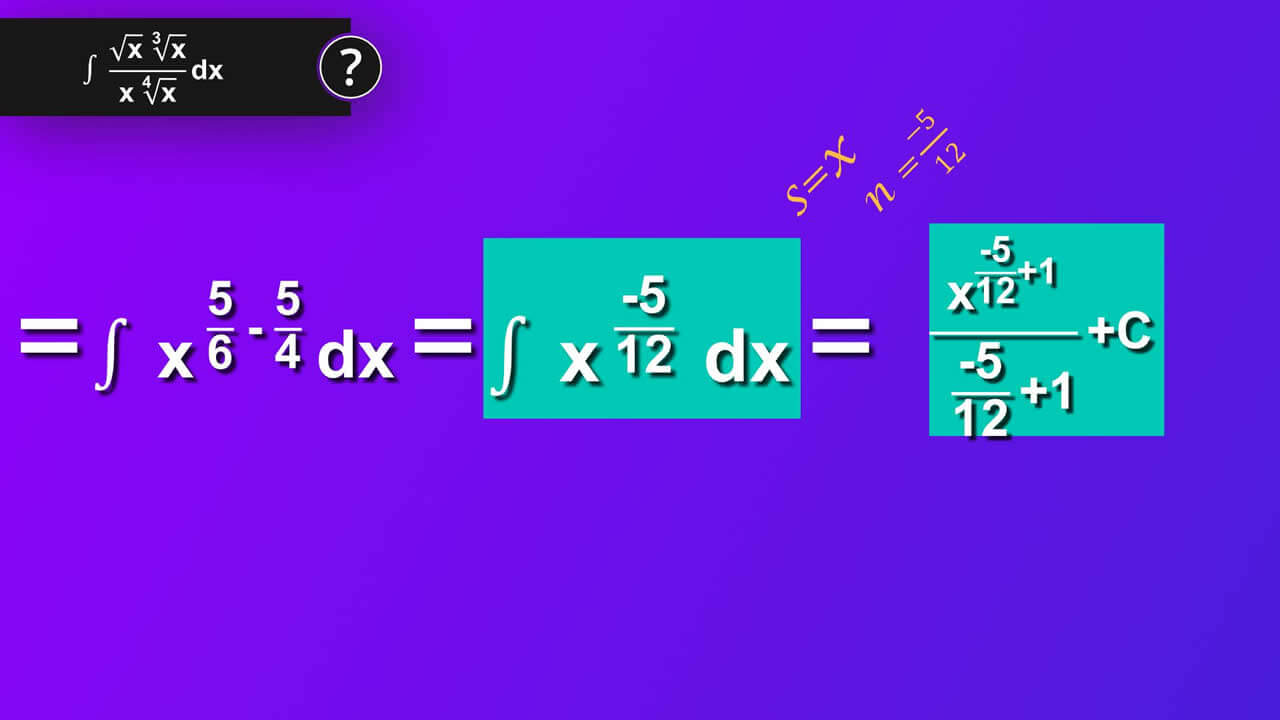
Step 9
We’ve got x rise to minus five-twelfth plus 1 divided by minus five-twelfth plus one and plus constant C.
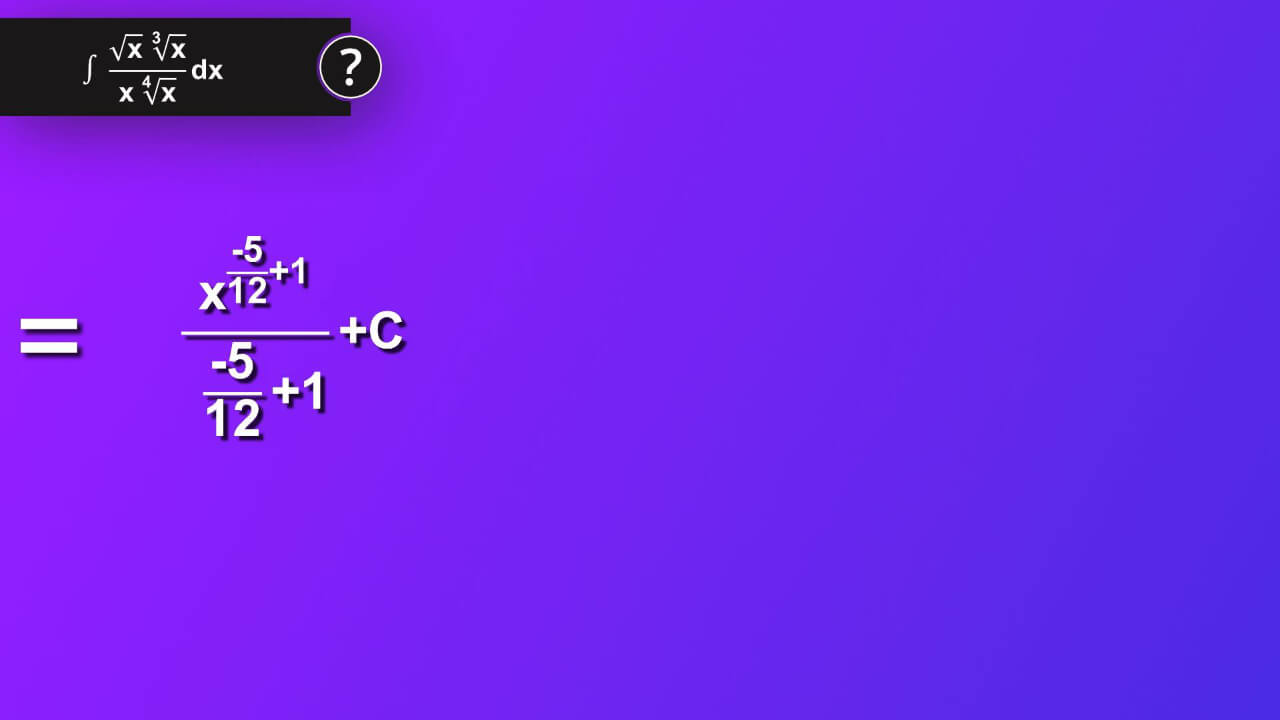
Step 10
First, let’s just add 1 to minus five-twelfth and we have minus five-twelfth plus twelve-twelfth, thus we’ve got seven- twelfth.
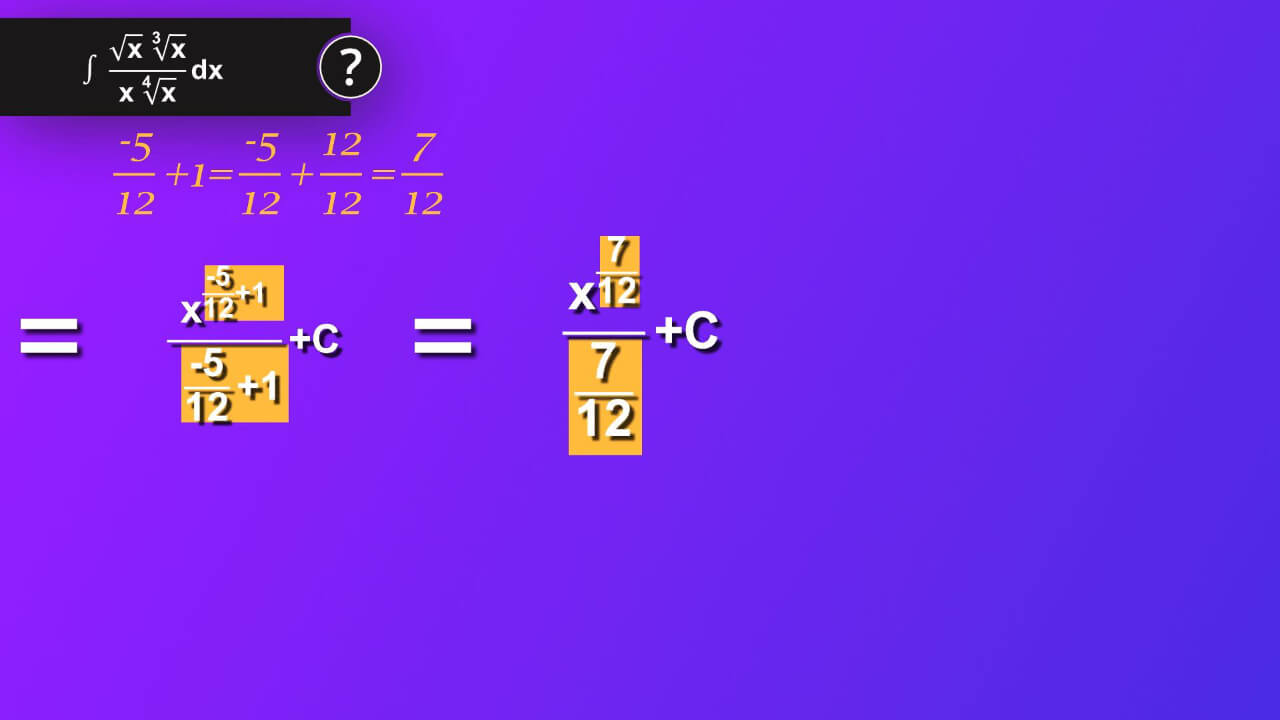
Step 11
We can insert it to power in numerator of our main solution and in denominator as well. We can smooth it a little taking advantage of formula which says that s divided by a divided by b equals b divided by a multiplied by s. In our case s is equal to x rise to seven-twelfth, a is 12, b is 7.
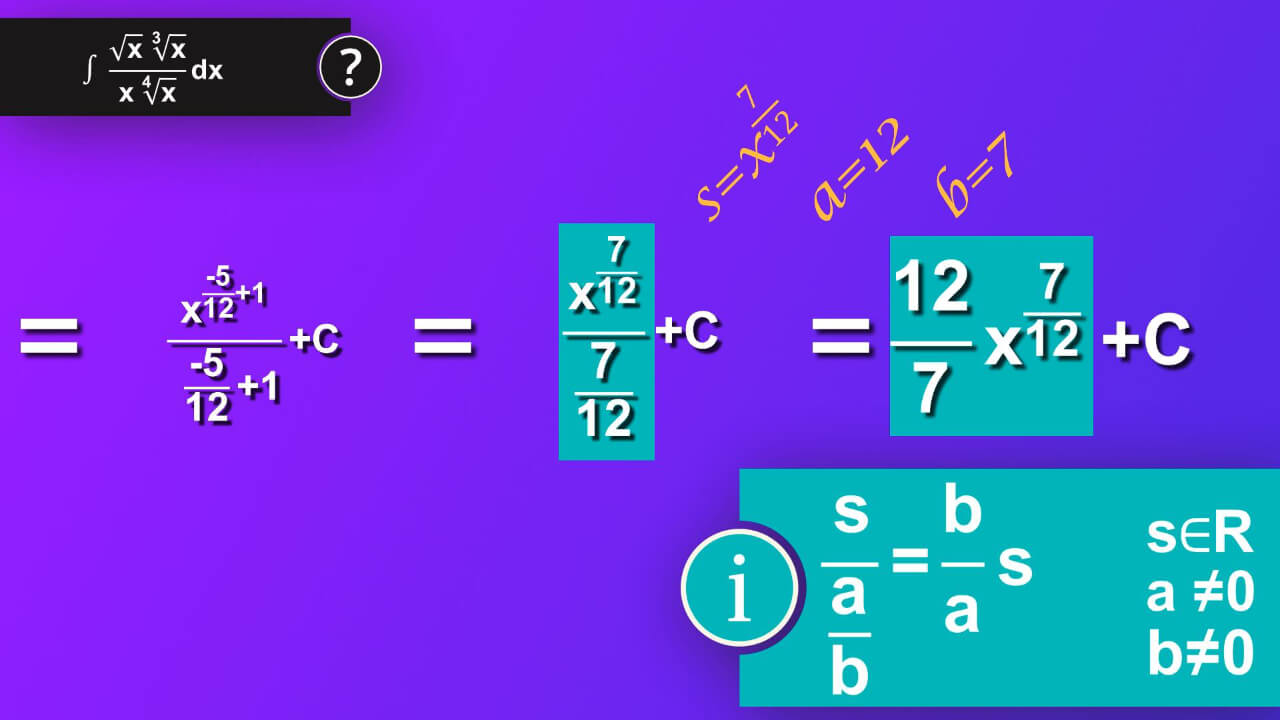
Step 12
And we have twelve -seventh multiplied by x rise to seven-twelfth plus constant C.
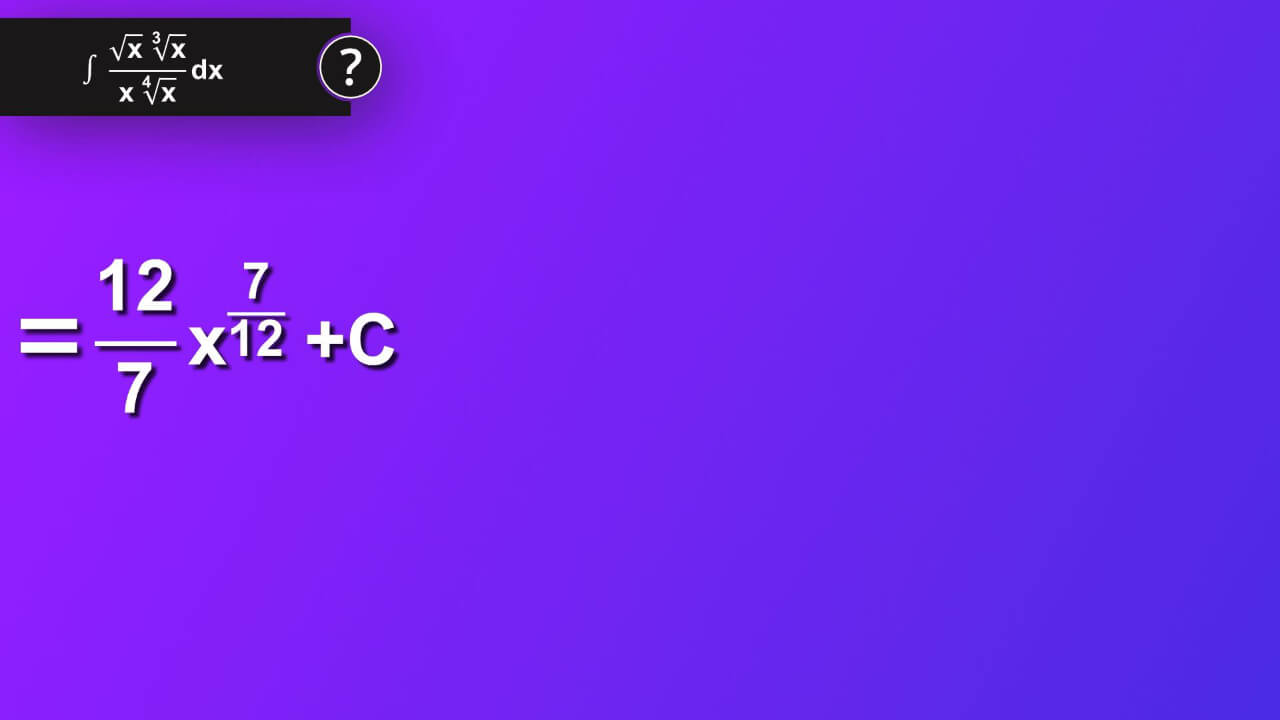
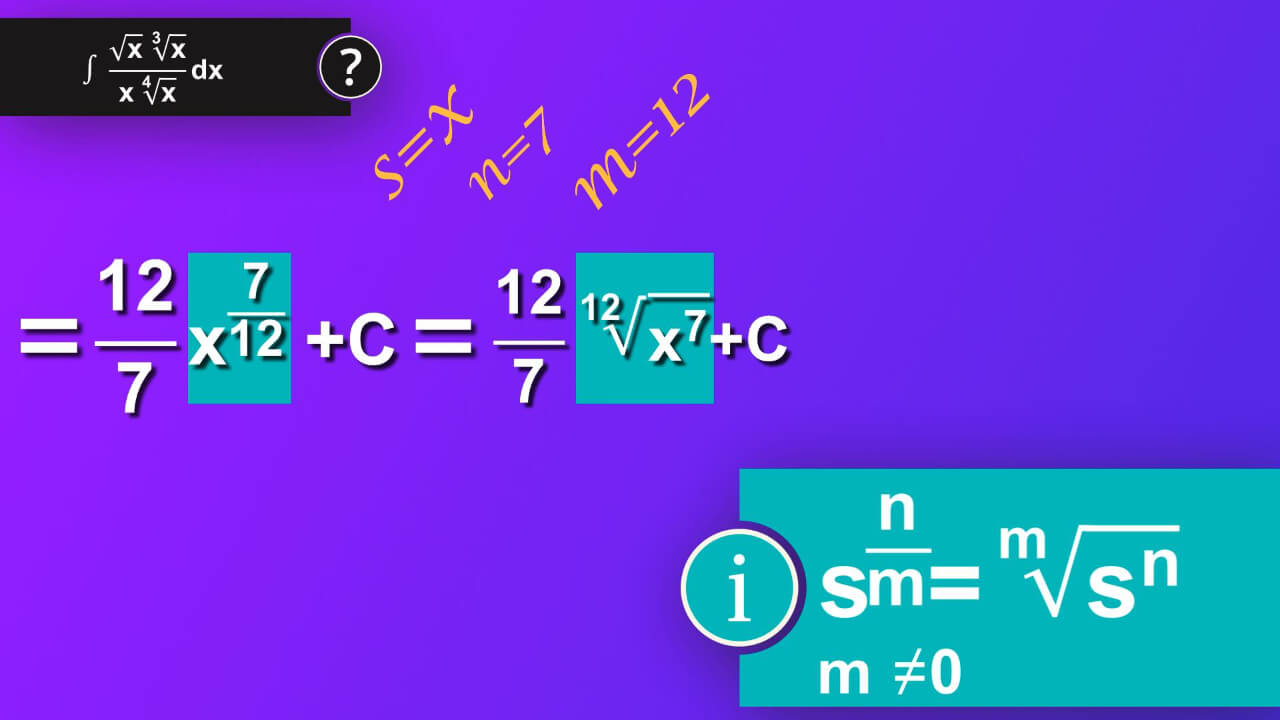
Step 13
We may also express differently our x rise to 7 divided by 12, knowing that s rise to n divided by m equals m-th root of s rise to n. In our case s is x, n is7, m is 12. As a result, we’ve got twelve -seventh multiplied by twelfth root of x rise to 7 plus constant C.
What is indefinite integral of square root of x multiplied by cube root of x divided by x multiplied by 4th root of x by dx?
We finally did it: ∫ ((√x∛x)/(x∜x)dx=(12/7)(12th root of x^7)+C
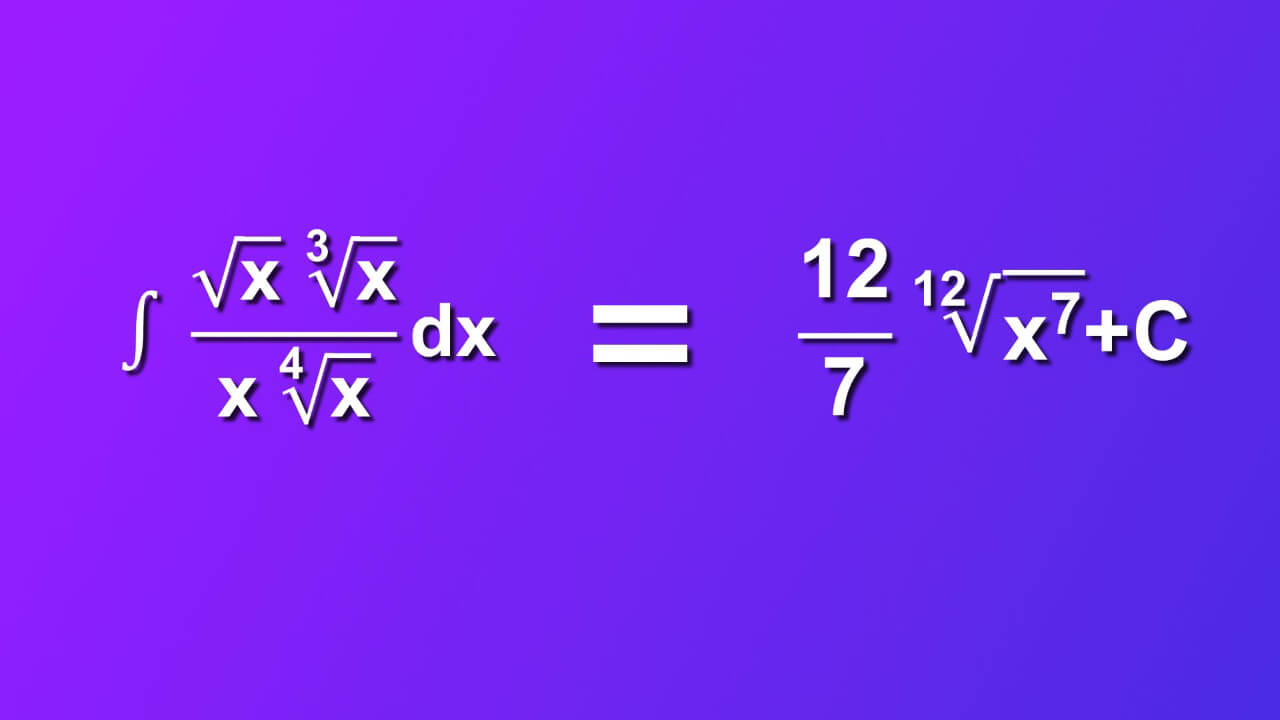
Dictionary
Integration (antidifferentiation)
Computation (process of finding) of an integral, opposite process to differentiation.Integrand
Function placed between sign of integral and differential of variable of integration e.g. $${{ \int f(x)dx}}$$,where:
$${{ \int }}$$- integration operator,
f(x) – integrand,
dx- differential of variable of integration x
Integrable function
Function that integral over its domain is finite.Indefinite integral
Represents a class of primitive functions whose derivative is the integrand e.g. $${{\int f(x)dx=F(x)+C \Leftrightarrow F’(x)=f(x)}}$$$${{C=const. }}$$,
$${{f, F,C \in R }}$$
R-real numbers
$${{\int f(x)dx}}$$ - indefinite integral of function f(x) by dx,
$${{F(x)+C}}$$ – a class of primitive functions that $${{F’(x)=f(x) }}$$,
F(x) - primitive function, usually written in capital letters,
R-real numbers.
Function
Function specified on a set X and having values in set Y is an assignment each element of set X specifically one element in set Y.$${{f: X \rightarrow Y}}$$
f-function name,
X-set of elements of function f, domain of a function f
Y-set of function values of function f, codomain of a function f
$${{x\in X, y \in Y}}$$
$${{y=f(x) f: x\rightarrow y}}$$
$${{y=y(x), }}$$
y(x)-vales of the function named y,
x-independent variable,
y-dependent variable.